What is Margin of Error?
To calculate the margin of error of your data sets, you need to ensure that the selected research sample truly represents your population of interest. This starts from identifying the right population of interest to applying the appropriate sampling technique. Before we get into it, let’s define what MOE is.
We can define margin of error (MOE) as the degree of error in results or the amount of random sampling error in the results of a survey. Alternatively, we can define it as a figure that tells you how near you can expect the survey results to be to the correct population value.
We use MOE when calculating the confidence interval. The margin of error of a data set directly influences the confidence level of the investigator in the research outcomes.
Typically, if your research outcome or survey results have a higher margin of error, it means that the data sets might be unreliable. On the other hand, a lower margin of error indicates that the results truly represent the research population.
Importance of Margin of Error.
- Margin of error helps you achieve reliable outcomes in research as it accounts for any disparities between results from the research sample and the target population.
- MOE helps researchers determine how representative the the ample is. A low margin of error shows that the sample population closely represents the population of interest in a systematic investigation.
- Margin of error helps you determine the confidence level and confidence interval of your data sets.
- Additionally, the margin of error helps researchers determine the accuracy of a value, by expressing it in a range.
How to Reduce the Margin of Error.
- Reduce the data variability.
- Enlarge your sample size.
- Use a lower confidence level.
- Implement a one-sided confidence interval.
Formula to Calculate Margin of Error.
To determine the margin of error;
- First calculate the σ standard deviation for your population and get a sample size.
- Take the standard deviation and divide it by the square root of your sample size.
- Multiple it with a z-score that is consistent with the confidence level, as per the following table:
| Percentage Confidence | Z-score |
| 80 | 1.28 |
| 90 | 1.645 |
| 95 | 1.96 |
| 98 | 2.33 |
| 99 | 2.58 |

Example:
Calculate the margin of error of a sample of 50 cones of ice cream, assuming you want a 99% level of confidence and the standard deviation is 3.
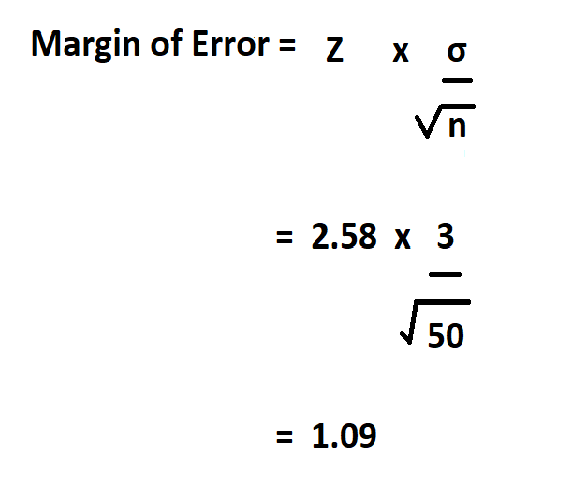
Therefore, the margin of error is 1.09.