What is an Equilateral triangle?
In this article, lets begin by defining what’s a triangle. A triangle is a polygon formed by three line segments linked end-to-end.
An equilateral triangle also known as an equiangular triangle is a type of a triangle that has three equal line segments or three equal sides.
Every equilateral triangle is also an isosceles triangle, as any two sides are equal and any two angles are also equal.
Properties of an equilateral triangle.
- It is a regular polygon with three sides.
- All the sides of an equilateral triangle are equal.
- All the three internal angles are congruent and equal to 60 degrees each, thus adding up to 180 degrees.
- The perpendicular drawn from vertex of the equilateral triangle to the opposite side bisects it into equal halves. Also, the angle of the vertex from where the perpendicular is drawn is divided into two equal angles, i.e. 30 degrees each.
- The ortho-centre and centroid are at the same point.
- In an equilateral triangle, median, angle bisector, and altitude for all sides are all the same
Formula to Calculate the Area of an Equiangular Triangle.
When you’re asked to calculate the area of an equilateral triangle you are simply being asked to find the region occupied by it in a two-dimensional plane. The formula for the area of an equiangular triangle is given by:

Example 1:
Calculate the area of the following equilateral triangle.

Therefore, the area of the equilateral is 10.83 cm² .
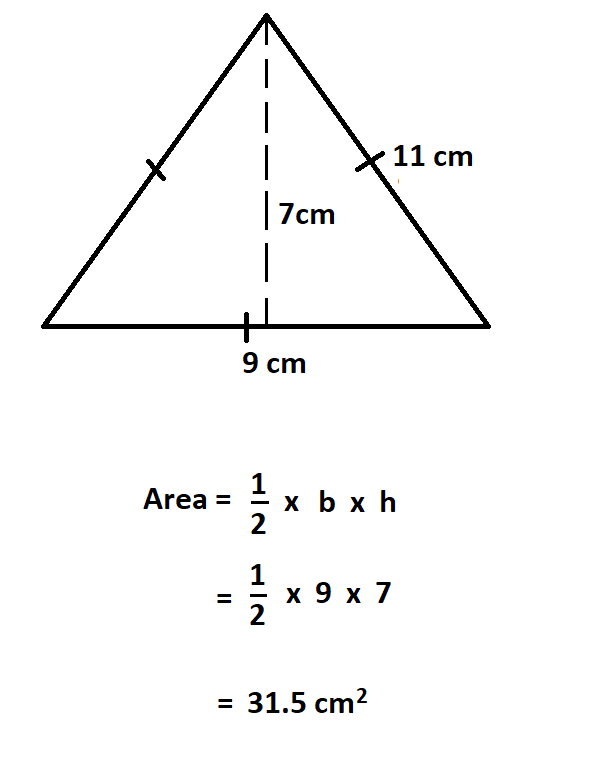
Example 2:
In an instance where you have been given the height of your equiangular triangle, you can just use the formula to calculate the area of a triangle.
For instance, calculate the area of the equilateral triangle below.